It occurred to be relatively early in during the trimester this past fall that my college algebra students (generally) have no idea what I mean when I say “quadratic function.”  This isn’t because they have never heard it, learned it, or used it.  But that technical of a term simply has not stuck around in their long term memory.

So, similar to linear functions, we start with a pattern:

I then had them make posters including how it is growing, what the 10th, 100th, 0th, and -1st cases would look like, table, graph, expression, and relationship to the pattern.  Instead of one large poster, they use 4 smaller pieces of paper and tape them together.  That way each group member can contribute simultaneously.

I noticed:

• It is difficult for students to describe how an irregular shape is growing.
• It is even more difficult for them to describe something abstract like the -1 case.
• Many of them expressed the overall growth as “exponential.”
• Most could easily see the two rectangles formed and determine the dimensions with respect to “n.”

I wondered:

• If they could connect the work with patterns to other quadratics.
• How to have a meaningful discussion around the “exponential growth” issue.

Their homework was to answer similar questions for this pattern from You Cubed’s Week of Inspirational Math:

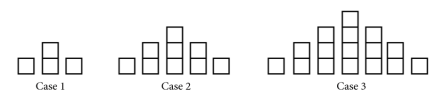
Spoiler alert: the rule for the pattern is f(n) = (x + 1)^2 or f(n) = x^2 + 2x + 1

So where do we go from here, two days before Winter Break?  My goals are to review some specifics on quadratic functions and simultaneously help the students make connections between different representations.  I know what I must do.  I must channel my inner Triangleman.

[Backstory:  Christopher Danielson and I go way back. At least to 2014. Maybe even 2013.  Seriously though, I strive to organize my college algebra class the way Professor Danielson describes in his blog.  I have picked his brain on more than a few occasions and he is gracious enough to give me advice in certain curricular areas. In short, his philosophy titled “They’ll Need it for Calculus” is the foundation of my College Algebra course. ]

Ok, back to room C118.

Me: Write down everything you know about the function y = (x+1)^2

(Most write down the expanded form, some start to graph, but not many)

Me: What other ways can we represent this function?

Students: Tables! Graphs! Pictures! Words! Patterns! Licorice!

Me: Sweet!  Let’s do all of that, minus the Licorice.

(I give them a few minutes to create a table and a graph.)

Me: NOW, write down everything you know about this function.

I circulate and hand each group a half sheet of paper.

Me: Write down the most important thing on your groups list.

At first I wasn’t really concerned what exactly they wrote down, but how they defended their choice. Then I came across this in all of my classes:

We came up with a pleasing list of attributes of a positive parabola that included vertex placement, end behavior, leading coefficients, and rate of change.

Next up for discussion: Parabolas grow exponentially.

Me: Turn to your partner and tell them whether you agree or disagree with this statement and defend your choice.

Students: Yes, words, words, words.

Me: Ok, now the other partner, say how you know something grows exponentially.

Students: Multiplied every time, more words, blah blah blah.

So we agreed that 2, 4, 8, 16, 32, 64… is an example of something that grows exponentially.

Me: Numerically, how can we tell how something is growing?

Students: (eventually) Rate of Change!

We came up with this table and agreed that these two functions were definitely NOT growing in a similar way.

Now on to helping them understand what it means for something to grow quadratically…