Month: January 2016

Watching Solitaire in Silence

Remember Windows Solitaire? I have fond memories playing this fantastic digital distracter with my high school beau on his brand new Gateway computer.  We would take turns striving for success in this card-clicking frenzy, the other watching and waiting patiently for the deck to empty.

But have you ever watched someone play solitaire on the computer?  It is so…what word comes to mind?  Frustrating?  Infuriating?  Aggravating, perhaps?  And why is that?

Check out this screenshot:

solitaire

What if the player was about to click on that blue, flowery deck of cards…would you be fighting the urge to save them from their potentially game-ending error of failing to move the sequence beginning with the six of spades to its rightful place atop the seven of hearts?  Or would you idly sit by and let them to figure out that solitaire is won by carefully searching for card moves before drawing from the deck?  Would you make any suggestions for improving their game once failure was inevitable?

I think this solitaire analogy is a lot like teaching.   I realized fully today why the “productive struggle” is so hard to sustain and perhaps why teachers so often fall back on traditional methods of delivering information to students:  Watching people struggle without intervening is difficult. Just as it’s natural to want to smooth out the path for our children, it’s also tempting to do the same for our students.  It’s just easier (and so much faster) to zip Maria’s (my daughter) coat or buckle her seat belt or pick up her toys.

As a simple, mathematical example, imagine one of your students is attempting to solve a quadratic equation. They start off like this:0126161730-1.jpg

Being the savvy algebra teacher you are, you can anticipate the error that the student is most likely going to make.  You’ve seen it hundreds, if not thousands of times.  Your inner teacher voice might be thinking, “For the love of humanity, Herbie (not your real name), set the dang thing equal to zero!  Quadratic formula!  IT’S GOT A SONG, FOR GOODNESS SAKE!”

Instead, you do not impede their solving and let them continue on their merry, algebraic way. 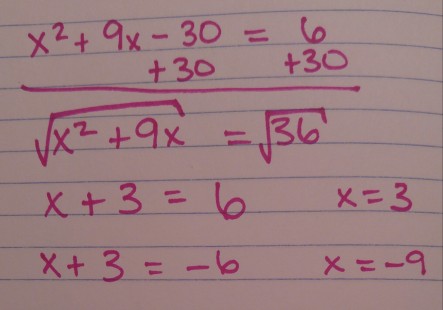 0126161732-2.jpg

Re-enter teacher voice in your head, “Now look what you did, Herbie.  You’ve gone and…wait…one of those answers is right.  Great.  Now we’ve really got issues.”

So what do we do about this?  Clearly the student needs some redirection and the teacher’s role is to guide the learning.  But had we intervened during earlier steps, we rob this student of a golden opportunity for brain growth.  Plus, we deprive the rest of the class the chance to learn from the misconception.  Even more, what a fantastic extension we have here:  why did the student get part of the problem correct and part incorrect?

In summary, we deny students the opportunity to learn from mistakes if we  prevent them from making mistakes in the first place.

Related Side Note:  I’m currently reading The Gift of Failure by Jessica Lahey.  Her introduction about her son’s shoelace-tying trials seems strikingly familiar.  And I can use this antedote as a reminder when encountering the zippers, and the seat belts, in addition to quadratic equations.

 

 

5 CommentsLeave a comment

This is Our Theorem – College Algebra

“We came up with a theorem once at my old school.  The teacher has it in a frame behind his desk.”

This statement from one of my college algebra students made me both elated and sad at the same time.  Thrilled because this is the type of mathematics I believe all students should have the chance to engage in on a regular basis.  Disappointed because this type of discovery happens so infrequently in American mathematics classrooms that the incident warranted a sacred place on the wall of this teacher’s room.

In College Algebra, part of today’s learning objective was to define a polynomial function and determine some key features.  I have the awesome types of students that if I were to write down the surly definition and features of a polynomial function onto the whiteboard, each would follow in lock-step and write it in their notebooks solidifying it’s place among mathematical obscurity.

Today, we were going to break that cycle with something different.

But I needed to know where they were at, so I had them write down what they knew about a polynomial function.

0125161447-1.jpg0125161445-1.jpg

After some discussion and leading questions, we were sure that linear, quadratic, cubic, quartic, x^5, x^6, and so on were all polynomial functions.  Awesome. We weren’t, however, as sure about functions including negative exponents, roots, sin/cos, or algebraic fractions.

What makes this group we are sure about special?  Last week, we spent a considerable amount of time on features of functions including domains, end behavior, intercepts, intervals, symmetry, and turning points.  In their groups, I had them examine the graphs of these alleged “polynomials” through the lens of the features of functions.

Two similarities emerged as significant:

0125161453-1.jpg

Questions:  Was this true of all polynomial functions?  And if both conditions were not met, could we exclude it from our known polynomial functions?  Hiding my initial excitement, I then had them look at our list of “questionable” functions. For example, did “y = 9 + 1/x” meet each of these two criteria?

Christopher Danielson suggested that my class give this new theorem a name, so we could refer back to it with ease:

“Class. We have found that all polynomials blah blah blah…” [while writing the statement of the theorem on the board.]  In mathematics, when we have an important finding like this, and when all mathematicians have agreed the finding is true, it gets a name.  Sometimes it is named for a person, such as ‘Fermat’s Last Theorem’; sometimes it is named for what it says, as in ‘The Triangle Inequality’.  But that name makes it possible to refer to it going forward. It helps us to remember and to use the thing we figured out. So we need to name our theorem. Who has a name they’d like to suggest?”

Alas, the excitement of naming the theorem will have to wait until tomorrow.

1 CommentLeave a comment

Conceptual Function Foundation Follow-up

One of my favorite parts about teaching is having the opportunity to see growth in myself and my students.  I love when a lesson I have used with success previously gets even better the next time around, especially when it is a lesson that exemplifies my teaching philosophy.

Last trimester, I used the New Visions for Public Schools’ Algebra 2 functions unit to help build a conceptual foundation.  I wrote about that experience here.

Day 1:  To start off this unit on functions, they were in pairs:  one partner facing the projector screen and one facing the back of the room.  I then drew an arbitrary function on an unlabeled set of axes.  The person facing the screen needed to use words only (no pointing, no gestures) to help their partner draw the graph.  The person drawing was not allowed to ask questions, just draw what they hear.  For example:

0113160857.jpg 0113160857a-1.jpg

Follow-up question (before they turn and look): What could you tell your partner to help them improve their graph?

Afterward, we talked about what descriptions were helpful.  My goal was to turn those descriptors such as “hills” and “curvy lines” into more specific function features.  Classwork (with their group of 4) involved looking at specific graphical examples to define end behavior, turning points, positive and negative intervals, etc.

Day 2:  Gave students a set of 36 graphs.  They needed to sort the graphs into exactly four groups based on their function feature.  I then followed up by having them choose one graph that best represented each group.  Some examples of student work:

0115160837-1.jpg 0115160837a-1.jpg 0115160838-1.jpg 0115160838a-1.jpg

Next week, we will look at their groupings and decide which ones highlight important features of the graphs.  Then, we will see if we can add some specificity and some real-world.  Seriously, I love the way this progression helps my students make sense of the function features.  It sure beats standing at the front and going through examples.

 

0 CommentsLeave a comment

Use All the Methods!

 

This is from Illustrative Mathematics (the people over there do wonderful work.  Plus they are lovely.) The problem I posed to my college algebra class was this:

Capture

I had them try it on their own and as I circulated the room, I noticed about 3 methods:  taking the square root, putting the problem in standard form and then factoring, or putting in standard form then applying the quadratic formula.  After clearing up errors and misconceptions, I was confident we understood that the answers were x = 3 and x = 9.

What now?  We could gather up all of the methods they came up with and make a lovely list.  Or we could take a look at the method that students almost 100% of the time ignore/forget/dismiss:  graphing.

Step 1:  Understand what a “solution” looks like graphically.

0108161549-1.jpg

We separated the equation into two quadratic functions which both were equal to y.  Now we had a system of equations and this group knows that systems of equations have solutions at points of intersection.

 

 

 

 

 

 

Step 2:  Without a graphing calculator, sketch each of these two functions to approximate how they cross. “Expect to be wrong and give it a go anyway so that we can all learn from each other.”

Step 3: Examine some of our solutions.  As I expected, only about 2 students had a solid understanding of where y = (2x-9)^2 sat on the xy-plane.

I categorized the errors into three groups:

A.  The negative 9 means the graph is shifted down.  0108161551-1-1.jpg

B.  Our answers when we solved were x = 3 and x = 9, so this graph must cross there. 0108161551-1-2.jpg

 

C.  I don’t want to be wrong so I’m only graphing y = x^2. 0108161551-1-3.jpg

Step 4: Look at the graphs of some similar quadratics like y=(x – 3)^2 and see if that thinking applies here.

*I feel like here is where understanding happened.

Step 5:  Take the gridlines and axes off of the Desmos graphs and find the points of intersection.

Capture

Interesting to see them “know” that x=3 and x = 9 from solving this equation algebraically somehow applied here, but they weren’t sure how.

Eventually we arrived at (3,9) and (9,81).

My Take-Away(s)

We explored a method that most (if not all) students don’t think of when asked to solve an equation:  Graphically.  But I think it’s an important one when trying to figure out how the pieces of functions and algebra fit together.  Yes, we could practice factoring, the quadratic formula, and completing the square all day long.  But in the end, know those individual methods doesn’t give my students an idea of how those solutions connect with the actual functions they represent.

 

This problem made me think about what we tell students when we explain methods of solving equations.  Any time we show a student a method, we are inexplicitly stating that this method has higher status than any other.  Giving students an opportunity to solve a problem using their prior knowledge is important to the learning process.   Their way of solving isn’t always going to be algebraic and building from where they are at is vital to creating a foundation of understanding.  If they start with “guess and check,” help them build structure from that rather than insist that the algebraic method of solving is superior.  In the case of the Problem above, any algebraic method was probably the most efficient but it isn’t always.

For example, what about this problem from Nrich.  Would your students approach it algebraically first?  Or is there foundation elsewhere?

Capture2

 

2 CommentsLeave a comment