“Mommy, will you play school with me?” Those words usually send me into an anxious panic given that I spend all of my working hours “playing school.” But now that I am on a leave of absence from my regular teaching gig, I’ve been able to play school with my daughter with calm humility.

Sidebar: If you want to know what really goes on at your child’s school, ask them to play school with you. It’s fascinating.

Anyway, so a few nights ago, Maria lays this gem on me: “Mommy, do you want to play school? I want to learn about fractions.” I have never dropped what I was doing so quickly to go play school. Fractions. YES!

Oh, wait. No. I don’t know how to teach fractions. Especially foundational work on fractions. I mean, yes, I know how to compare fractions, I know where they are on the number line, I know the algorithms for fraction operations and I know how they work for the most part. But helping my 7-yr old develop a conceptual understanding of a fraction. Nope. One thing I was certain about: I needed to bust out the pattern blocks.

I’ve heard many a secondary teacher complain about how the kids don’t understand fractions and don’t remember the rules for operations with fractions from 3-5th grades. Having recently taken a plunge into the world of discovering how an understanding of fractions is developed, two realizations emerged: 1. Teaching fractions from a conceptual framework with a classroom full of students is really REALLY difficult, complex work. 2. It isn’t surprising that kids don’t understand them very well given the constraints we have as math teachers (time, etc) to help develop that conceptual understanding.

Luckily, I have a lot of friends who have. “Start with asking her how she would share two cakes equally with four people.” I gave her two yellow hexagons (cakes). Because of her (always helpful) assistance in the kitchen, she knows about half in the sense that it divides a whole into 2 parts. So she quickly grabbed two of the red trapazoids and determined that we could divide the cakes in half with those.

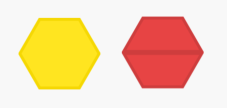
She did the same with the blue diamonds and green triangles for 3rds and 6ths.

Fast forward to last night. I’ve been reading Extending Children’s Mathematics and the first chapter outlines how kids begin their initial understanding of fractions based on equal sharing problems in 1st or 2nd grade. Knowing that Maria is always happy to do math if it means putting off bed time, I tried this problem with her:

Four children want to share 10 brownies so that everyone gets exactly the same amount. How much brownie can each child have?

She went through a number of strategies first determining that two per child was too small because there would be brownie leftover. Then she figured out that 3 per child would be too much because there wouldn’t be enough brownie.

M: It’s not possible to share 10 brownies fairly with 4 friends. Can we have 5 friends?

Me: Nope. These are BROWNIES. And we aren’t sharing this chocolate goodness with any more people.

M: Well, if each friend got two brownies, then there would be two leftover. (lots of thinking) Then we could split those two in half and each friend would get one of those halfs.

Me: Excellent strategy. So how much altogether would each friend get?

M: (It took my suggesting that she use her visual model to determine this) Two and a half brownies.

Me: Good. Now go brush your teeth and get ready for bed.

What I learned:

• Kids have an intuitive understanding of fractions that builds from their experiences with ‘fair sharing’ problems.
• The shift from working with whole numbers to working with fractions is a big one because of the variety of ways we use fractions (beyond part-whole).
• Helping kids develop a conceptual understanding of fractions is really hard work, and it’s really important for secondary teachers to learn more about the complexity of this work.